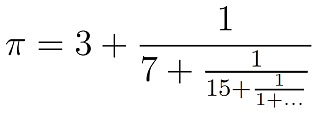Projekt: Algebra
Thema: Continued fractions
Gleb Pogudin
There are many different ways to speak about real numbers.
The most common way to write a real number down is to use decimal
fractions, for example: π = 3.1415 …
But the same number can be written in the form of continued fraction:
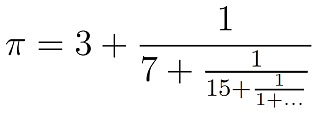
Usually continued fractions are used when you need to approximate something.
For example, the difference between π and its approximation
355 / 113
derived from the expression above is less than
1 / 1000000!
Moreover, continued fractions can explain the structure of calendar, help
to construct compact electric schemes, describe knotted molecules, and so
on.
During the project we will try to understand what they are good for, why
they are good, and how to use them in order to make the world a bit more
precise.
Abschlusspräsentation
der Gruppe Algebra

-->